Overview for Present Value of Annuity Due
Present value of annuity due table is a variable that calculates the annuity due. Therefore, in the following discussion, we will start a brief about annuity and the types of an annuity due further explaining the present value of annuity due table.
What is an Annuity?
An annuity can be described as a kind of multi-period investment where a principal amount is deposited and then regular payments are made during the phase of investment. The payments are of fixed size.
For example, a car loan can be an annuity where the company gives the person a loan to purchase a car. However, the individual makes an initial down payment and then each month a fixed amount. The sum of the payments made altogether will be greater than the loan amount, which explains an interest rate implicity charged on the loan. As a result, the payment schedules spread over time.
The advantages of making a loan an annuity can be:
- There is a regular cash flow which we know
- Predictable payments and returning the amount in smaller multiple periods is advantageous for an individual overpaying the whole lot at once.
- The institution can monitor the financial health of the customers or clients
Types of Annuity
Since annuities spread over multiple periods, there are different types of annuities relating to when the payments occur in the period. Following are the three types:
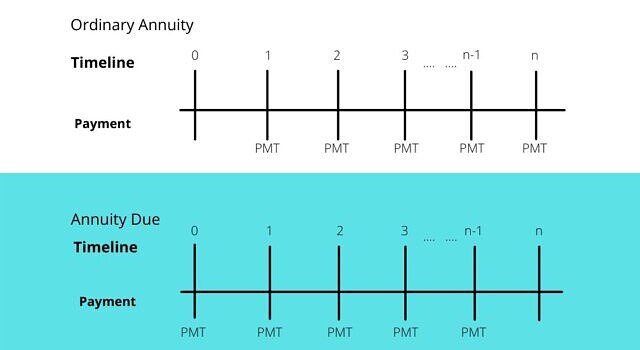
- Annuity due: Here the payments are made by the client at the start or beginning of the period. Like if the period is each month, the payments occur at the starting of each month.
- Ordinary Annuity: In this method, the payments occur at the end of each period. Like if the period is one month, this explains that payment is done on the 28th, 30th or 31st of each month depending on the type of month. This must be noted that mortgage payment is mostly ordinary annuities.
- Perpetuities: This explains that the payments continue forever. However, this condition is rarer than the first two types.
Annuity Due
This method describes the kind of annuity whose payment gets due at the beginning of the period immediately.
One common example of an annuity due can be rent since landlords often demand the payment at the start of a new month. Instead of collecting it after the guest has enjoyed the benefits of the apartment.
However, the future and the present value of annuity due table formulas differ slightly from ordinary annuity due to the differences in when the payments are made.
Calculating Annuity Due
The annuity due payments represent an asset received legally by an individual. However, the person paying the due has the debt liability needing periodic payments.
Since the annuity due payments present future cash outflows or inflows, the payer of the funds can calculate the entire amount of the annuity while factoring in the time value of money. This accomplishes by considering the future and the present value of annuity due table.
Note: The income from annuity gets taxed as ordinary income.
Insurance companies sold annuity due as a financial product that needs annuity payments to be made at the start of each month, quarter or annual period. This kind of annuity provides the companies during the distribution period with payments as long as the annuitant lives. However, as the individual passes, the insurance company retains the funds remaining.
Examples of Annuity due
The recurring obligation may lead to annuity due.
- Therefore, the monthly bills like car payments, mortgages, cell phone payments and rent, are few examples since the beneficiary needs to pay at the start of the billing period.
- As discussed above, insurance expenses as also annuities due as the insurer gets paid at the start of the coverage period.
- Also, annuity due situations may arise when the annuitant saves for retirement or put their money aside for a specific reason.
Present Value of Annuity Due
The annuity due can be calculated keeping the time value of the money in mind. One way is the present value of the annuity due table. It is used to derive the current value of cash payments that are to be made in predetermined amounts on predetermined future dates.
Also, It uses the basic present value of annuity due table concept for annuities, except that cash flows are discounted to time zero. The calculation is made to decide either an individual should take a lump-sum payment or a series of cash payments in the future.
So, The present value is calculated with the discount rate, which is nearly equal to the current rate of return on the investment. Therefore, This must be noted that the higher the discount rate, the lower will be the present value of the annuity due table and vice versa.
Time Value of money
The present value of annuity due table is a difficult topic to discuss since it relates to the topic of the time value of money. Time value of money explains that if an individual is given $1 today, its worth is more than the same $1 from five years now. This happens due to inflation ( price Level accounting) and the changing value of money along with its potential to earn interest.
Therefore, the present value of annuity due table refers to calculating the value at the end of given periods using the current value of money. Another way it can explain is how much an annuity due will be worth when the payments get complete in future, compared to the present.
Formula
The formula to find the present value of annuity due table where the payments are made at the start of each period is:
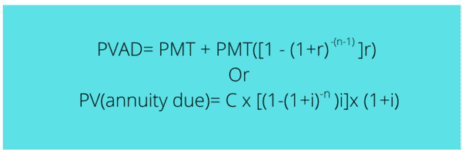
Where PMT = each annuity payment amount
r = interest rate
n = number of periods over which payments occurs
PVAD = present value of annuity due
Also, the same formula is used for the present value of an ordinary annuity where payments occur at the last of each period. Except that the extreme right side adds an extra payment. This explains when each payment occurs one period sooner than under an ordinary annuity.
Therefore, The factor used to calculate the present value is derived from the present value of annuity due table that lays out applicable factors by interest rate and the period in a matrix.
Present Value of Annuity Due Table
The present value of annuity due table is used as a quick reference to find the present value annuities. Also, It provides the figures for interest rates and discrete-time periods that may not correspond every time to the real world. Therefore, there are different formulas available for each kind. So, Few interest rates are as below:
| n | 1% | 2% | 3% | 4% | 5% | 6% | 8% |
| 1 | 1.0000 | 1.0000 | 1.0000 | 1.0000 | 1.0000 | 1.0000 | 1.0000 |
| 2 | 1.9901 | 1.9804 | 1.9709 | 1.9615 | 1.9524 | 1.9434 | 1.9259 |
| 3 | 2.9704 | 2.9416 | 2.9135 | 2.8861 | 2.8594 | 2.8334 | 2.7833 |
| 4 | 3.9410 | 3.8839 | 3.8286 | 3.7751 | 3.7232 | 3.6730 | 3.5771 |
| 5 | 4.9020 | 4.8077 | 4.7171 | 4.6299 | 4.5460 | 4.4651 | 4.3121 |
Example for Present Value of annuity due
For Example 1: To calculate the present value of annuity due table of future rent payments specified in the lease. Suppose an individual pays $1000 per month as rent. Therefore, below is an explanation of what it will cost the person for the next five months, in terms of the present value with 5 % interest.

So, using first formula PV(annuity due)= 1000x 4.33 x (1+0.05)
=$4545.95
Example 2
XYZ International paid a third party $100 at the start of each year for the upcoming three years for rights to a key patent. So, Find the cost to XYZ, if it has to pay the whole amount with an interest rate of 5%.
P= $285.94
Therefore, $285.94 refers to the current value of $100 for three payments with a rate of 5%.
Future Value of Annuity Due
The future value refers to the total of all payments of the future values in the annuity. Therefore, it explains that it is possible to add up all future value of cash flows but not pragmatic if more than a couple of payments are there.
If the future value of all payments finds manually, then the explicitly about termination of annuity and inception is important. In the case of an annuity due since the payments occur at the start of each period, so the first payment is at the inception of the annuity while the last occurs one period before the termination.
Formula
Therefore to calculate the future value of the annuity more easily, we have a formula. For which we need to know the:
- An interest rate of the account the payments deposits depict by “r” or “i”
- Payment amount depicted by “C”, “pmt” or “p”
- Number of periods per year known by “n”
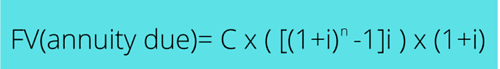
Example
This method results in higher values taking into account payments occurring at beginning of each period. The reason why values are higher can be explained that the beginning period payment leads to more time to earn interest.
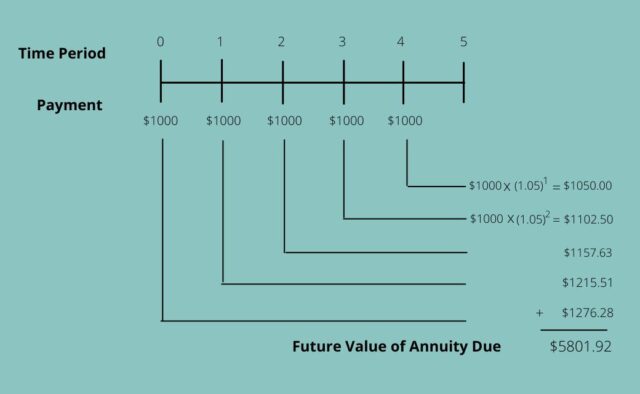
In Example 1 discussed above, using formula
FV(annuity due)= 1000 x 5.53 x (1+0.05)
=$5801.92
Therefore, The above example explains that the amount of interest gathered on the first $1000 receives interest for 5 years making it $1276.28. While the last instalment receives, the total interest of $1050.00 only since it deposits for one year.
Conclusion
Therefore, the present value of annuity due table explains an easier way to find the values. An individual can use spreadsheets instead of formulas if he does not remember.
Moreover, there are two types of annuities as annuity due and ordinary annuity which can further divide to present and future values. This explains that keeping in mind the time value of money, any figure can calculate, either the payment is at the beginning or end.